Sierpinski Triangle

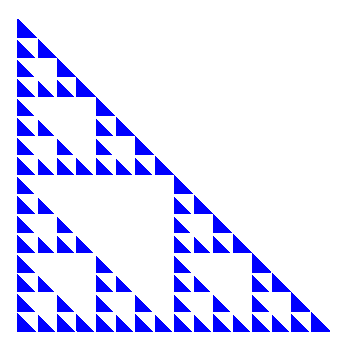
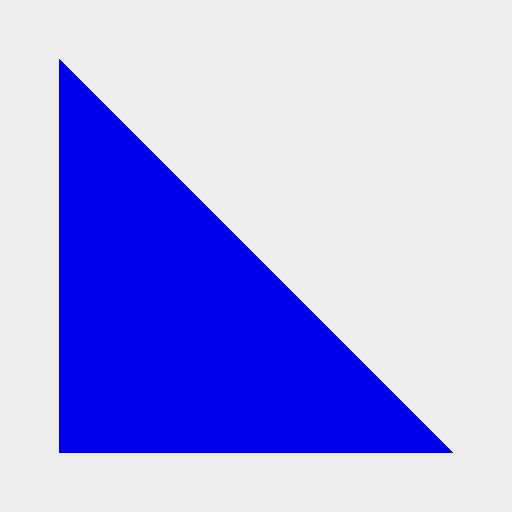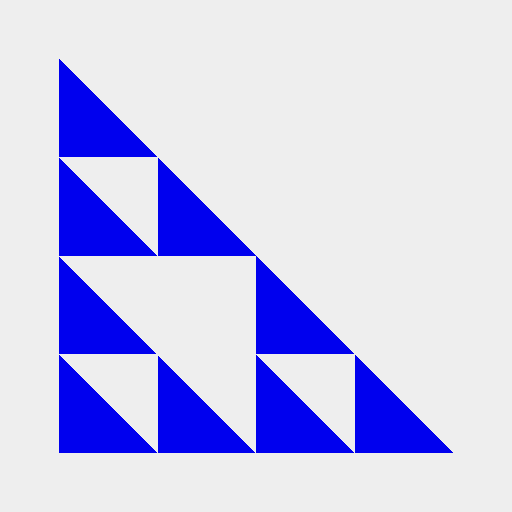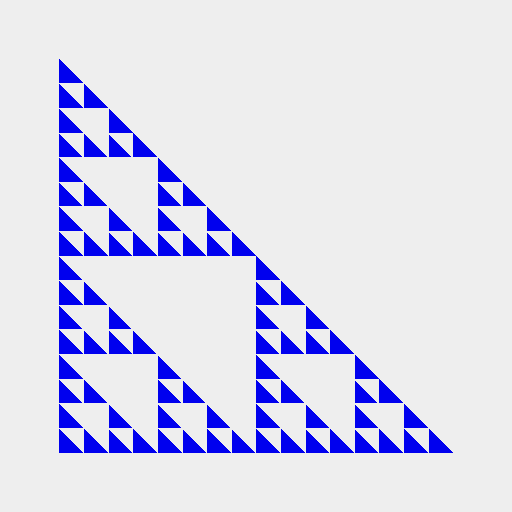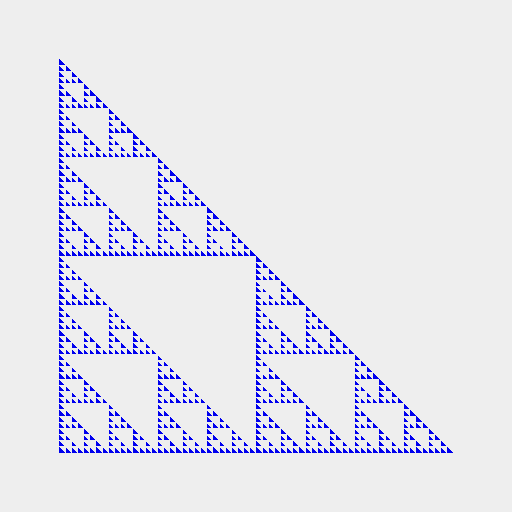
The Sierpinski Triangle is obtained as the residual set remaining when one begins with a triangle and applies the operation of dividing it into four equal triangles and omitting the interior of the center one, then repeats this operation on each of the surviving 3 triangles, then repeats again on the surviving 9 triangles, and so on $\cdots$ . See [36, Example 2.7].

The Sierpinski Triangle is homeomorphic to the unique nonempty compact set K of the complex plane that satisfies

$$K= w_1(K) \cup w_2(K) \cup w_3(K) \quad ,$$

where w₁, w₂, w₃ are maps of the complex plane defined by w₁(z)=z/2, w₂=(z + 1)/2 and w₃=(z+i)/2.

Sierpinski Triangle- fig. a


Sierpinski Triangle- fig. b


Source files: a.eps . a.gif . b.gif . example.htm . figure.mws . latex.tex . title.txt .

Here you can read Notes or write to Notes.